TM 1-1500-204-23-2
(a)
Force computation. The force (F) in pounds can be obtained by multiplying the known values of
area (A) and pressure (P).
(b)
Pressure computation. Similiary, if the force (F) and area (A) are known, F can be divided by A
to obtain pressure (P) In pounds per square inch.
(c)
Area computation. Finally, to obtain area In square inches, force (F) is divided by pressure (P)
c.
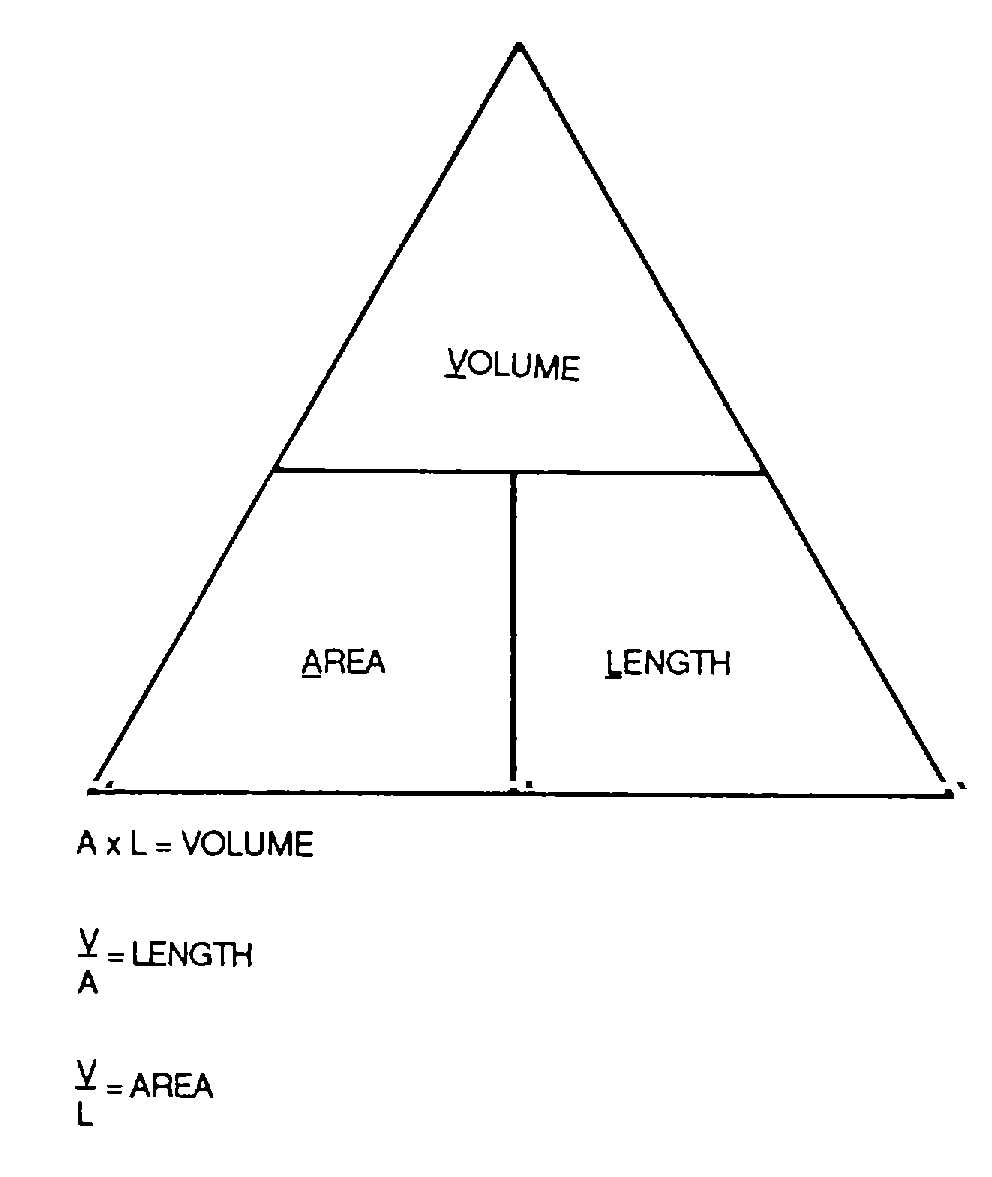
Computation of Volume, Area, and Length of Stroke. You can use the same type of triangular equation to
compute volume, area, and length of stroke. The following definitions apply for this manual.
(1)
Volume. Volume is a measurement of quantity expressed in cubic inches, for example, the amount of
liquid in a cylinder or the amount of liquid displaced by a pump or an actuating cylinder.
(2)
Area. As in the previous equation, area is a surface measurement expressed in square inches.
(3)
Length of stroke. Length of stroke is a measurement of distance expressed in inches. The stroke
length with which you will be concerned in this manual is the distance a piston moves in a cylinder.
(4)
Computation. If you know any two of these factors, you can compute the third (see figure 2-2). As in
the previous equation, multiply the two lower factors together to obtain the top factor and divide the top factor by the
known lower factor to obtain the unknown lower factor. For example, a piston having an area of 8 square inches moves
a distance of 10 inches within a cylinder. To find the volume of liquid moved, multiply the two lower factors together-8
square inches (A) times 10 inches (L)- to obtain V: 80 cubic inches
d.
Pascal's Law
. Practical applications of hydraulic principles are based on Pascal's law, which may be stated
in simple terms as follows: when a force is applied to a confined fluid, the pressure is transmitted equally in all
directions. Pascal's law applies for confined fluids only. It follows then that the law does not apply for fluids in motion
since moving fluid is not confined in the true sense of the word. Figure 2-3 shows graphic illustration of how Pascal's law
applies in an aircraft hydraulic system. Note that when a force is applied to the piston in cylinder 1, it is transmitted to all
portions of the confined fluid. If, for example, the applied force is 5 psi, the pressure In cylinders 1, 2, and 3 and in the
tubing will be 5 psi. But suppose a change takes place in which a lack of resistance causes either piston 2 or 3, or both,
to move upward. This momentarily lowers the pressure in cylinders 2 and 3 while in cylinder 1 it is still 5 psi. This
unbalanced condition cannot last. Fluid will flow from cylinder 1 to cylinders 2 and 3. This is because pistons 2 and 3
are not confining the fluid as long as they move upward. Pascal's law does not apply to this condition. When pistons 2
and 3 reach the end of their strokes, the fluid stops flowing. At this point the fluid is again confined and the pressure
again equalizes-Pascal's law applies once more.
e.
Mechanical Advantage. In simple terms, mechanical advantage is defined as the use of a small force over a
great distance to obtain a great force over a short distance. In other words, it is the amount by which the input effort is
multiplied in the output of a device. Using a claw hammer to remove a nail from a two-by-four piece of lumber or using a
jack to raise a vehicle are examples of mechanical advantage. The aircraft hydraulic system Is designed to make full
use of this principle since it is a system for transmitting engine power to distant points in the aircraft where power is
needed. This is accomplished by multiplying the input effort (or force) enough to do the required job-in other words,
gaining a mechanical advantage.
Figure 2-2. Computing Volume, Length, and Area
2-2